Which Of The Following Best Describes The Difference Between Data Andã¢â‚¬â€¹ Information?
3. Derivatives
3.2 The Derivative equally a Function
Learning Objectives
- Define the derivative function of a given role.
- Graph a derivative office from the graph of a given office.
- State the connection between derivatives and continuity.
- Describe 3 conditions for when a part does not have a derivative.
- Explain the meaning of a college-order derivative.
As we have seen, the derivative of a office at a given point gives us the rate of change or slope of the tangent line to the function at that point. If we differentiate a position function at a given time, nosotros obtain the velocity at that time. Information technology seems reasonable to conclude that knowing the derivative of the part at every point would produce valuable information almost the behavior of the role. However, the process of finding the derivative at even a handful of values using the techniques of the preceding section would chop-chop become quite wearisome. In this section we define the derivative role and learn a process for finding it.
Derivative Functions
The derivative function gives the derivative of a function at each betoken in the domain of the original function for which the derivative is defined. We can formally define a derivative function every bit follows.
Definition
Let ![]() be a function. The derivative function, denoted past
be a function. The derivative function, denoted past ![]() , is the office whose domain consists of those values of
, is the office whose domain consists of those values of ![]() such that the following limit exists:
such that the following limit exists:
![]() .
.
A function ![]() is said to exist differentiable at
is said to exist differentiable at ![]() if
if
![]() exists. More by and large, a function is said to exist differentiable on
exists. More by and large, a function is said to exist differentiable on ![]() if it is differentiable at every bespeak in an open gear up
if it is differentiable at every bespeak in an open gear up ![]() , and a differentiable part is ane in which
, and a differentiable part is ane in which ![]() exists on its domain.
exists on its domain.
In the next few examples we use (Figure) to notice the derivative of a function.
Finding the Derivative of a Square-Root Part
Find the derivative of ![]() .
.
Solution
Start directly with the definition of the derivative role. Employ (Figure).
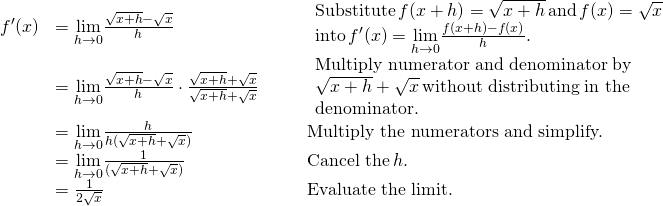
Finding the Derivative of a Quadratic Function
Discover the derivative of the function ![]() .
.
Solution
Follow the same process here, but without having to multiply by the cohabit.
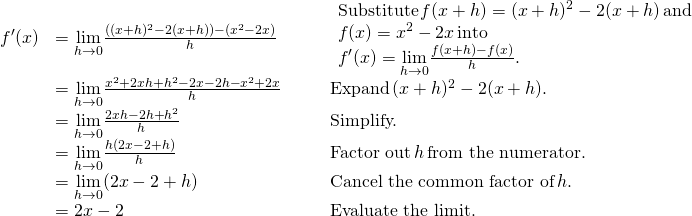
Find the derivative of ![]() .
.
Solution
![]()
We utilize a variety of unlike notations to express the derivative of a function. In (Effigy) we showed that if ![]() , and then
, and then ![]() . If we had expressed this function in the form
. If we had expressed this function in the form ![]() , we could have expressed the derivative as
, we could have expressed the derivative as ![]() or
or ![]() . We could accept conveyed the aforementioned information past writing
. We could accept conveyed the aforementioned information past writing ![]() . Thus, for the function
. Thus, for the function ![]() , each of the post-obit notations represents the derivative of
, each of the post-obit notations represents the derivative of ![]() :
:
![]() .
.
In place of ![]() we may also use
we may also use ![]() Utilize of the
Utilize of the ![]() notation (called Leibniz notation) is quite common in engineering and physics. To empathize this notation better, recall that the derivative of a office at a point is the limit of the slopes of secant lines as the secant lines approach the tangent line. The slopes of these secant lines are frequently expressed in the form
notation (called Leibniz notation) is quite common in engineering and physics. To empathize this notation better, recall that the derivative of a office at a point is the limit of the slopes of secant lines as the secant lines approach the tangent line. The slopes of these secant lines are frequently expressed in the form ![]() where
where ![]() is the difference in the
is the difference in the ![]() values corresponding to the departure in the
values corresponding to the departure in the ![]() values, which are expressed equally
values, which are expressed equally ![]() ((Figure)). Thus the derivative, which can exist idea of every bit the instantaneous rate of change of
((Figure)). Thus the derivative, which can exist idea of every bit the instantaneous rate of change of ![]() with respect to
with respect to ![]() , is expressed as
, is expressed as
![]() .
.
Graphing a Derivative
We accept already discussed how to graph a function, so given the equation of a function or the equation of a derivative function, we could graph it. Given both, we would expect to see a correspondence between the graphs of these two functions, since ![]() gives the rate of change of a function
gives the rate of change of a function ![]() (or gradient of the tangent line to
(or gradient of the tangent line to ![]() ).
).
In (Effigy) we found that for ![]() . If we graph these functions on the same axes, every bit in (Figure), we tin use the graphs to empathise the relationship betwixt these ii functions. Outset, we detect that
. If we graph these functions on the same axes, every bit in (Figure), we tin use the graphs to empathise the relationship betwixt these ii functions. Outset, we detect that ![]() is increasing over its entire domain, which ways that the slopes of its tangent lines at all points are positive. Consequently, nosotros expect
is increasing over its entire domain, which ways that the slopes of its tangent lines at all points are positive. Consequently, nosotros expect ![]() for all values of
for all values of ![]() in its domain. Furthermore, as
in its domain. Furthermore, as ![]() increases, the slopes of the tangent lines to
increases, the slopes of the tangent lines to ![]() are decreasing and nosotros expect to see a corresponding decrease in
are decreasing and nosotros expect to see a corresponding decrease in ![]() . Nosotros too observe that
. Nosotros too observe that ![]() is undefined and that
is undefined and that ![]() , corresponding to a vertical tangent to
, corresponding to a vertical tangent to ![]() at 0.
at 0.
In (Figure) we found that for ![]() . The graphs of these functions are shown in (Effigy). Notice that
. The graphs of these functions are shown in (Effigy). Notice that ![]() is decreasing for
is decreasing for ![]() . For these aforementioned values of
. For these aforementioned values of ![]() . For values of
. For values of ![]() is increasing and
is increasing and ![]() . Also,
. Also, ![]() has a horizontal tangent at
has a horizontal tangent at ![]() and
and ![]() .
.
Sketching a Derivative Using a Function
Use the post-obit graph of ![]() to sketch a graph of
to sketch a graph of ![]() .
.

Sketch the graph of ![]() . On what interval is the graph of
. On what interval is the graph of ![]() above the
above the ![]() -centrality?
-centrality?
Solution
![]()
Derivatives and Continuity
Now that we can graph a derivative, permit's examine the behavior of the graphs. First, we consider the human relationship between differentiability and continuity. We will see that if a part is differentiable at a point, it must be continuous at that place; however, a function that is continuous at a signal need non exist differentiable at that point. In fact, a function may be continuous at a indicate and fail to be differentiable at the point for one of several reasons.
Proof
If ![]() is differentiable at
is differentiable at ![]() , then
, then ![]() exists and
exists and
![]() .
.
We want to prove that ![]() is continuous at
is continuous at ![]() past showing that
past showing that ![]() . Thus,
. Thus,
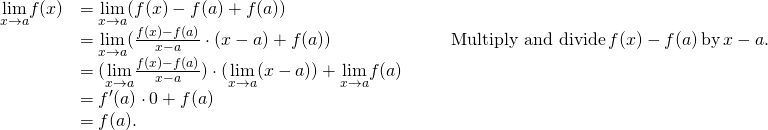
Therefore, since ![]() is defined and
is defined and ![]() , nosotros conclude that
, nosotros conclude that ![]() is continuous at
is continuous at ![]() .
. ![]()
We have merely proven that differentiability implies continuity, but now we consider whether continuity implies differentiability. To determine an answer to this question, we examine the part ![]() . This function is continuous everywhere; even so,
. This function is continuous everywhere; even so, ![]() is undefined. This observation leads the states to believe that continuity does non imply differentiability. Permit'south explore farther. For
is undefined. This observation leads the states to believe that continuity does non imply differentiability. Permit'south explore farther. For ![]() ,
,
![]() .
.
This limit does not exist because
![]() .
.
Run into (Figure).
Allow's consider some additional situations in which a continuous function fails to be differentiable. Consider the function ![]() :
:
![]() .
.
Thus ![]() does not exist. A quick wait at the graph of
does not exist. A quick wait at the graph of ![]() clarifies the situation. The function has a vertical tangent line at 0 ((Figure)).
clarifies the situation. The function has a vertical tangent line at 0 ((Figure)).
The function 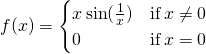 also has a derivative that exhibits interesting behavior at 0. We come across that
also has a derivative that exhibits interesting behavior at 0. We come across that
![]() .
.
This limit does not exist, substantially because the slopes of the secant lines continuously change management equally they arroyo zero ((Effigy)).
In summary:
- We notice that if a function is non continuous, it cannot be differentiable, since every differentiable function must be continuous. Nevertheless, if a function is continuous, it may nonetheless neglect to be differentiable.
- We saw that
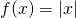 failed to be differentiable at 0 because the limit of the slopes of the tangent lines on the left and right were not the same. Visually, this resulted in a sharp corner on the graph of the function at 0. From this we conclude that in order to be differentiable at a point, a function must be "polish" at that point.
failed to be differentiable at 0 because the limit of the slopes of the tangent lines on the left and right were not the same. Visually, this resulted in a sharp corner on the graph of the function at 0. From this we conclude that in order to be differentiable at a point, a function must be "polish" at that point. - Every bit we saw in the example of
![Rendered by QuickLaTeX.com f(x)=\sqrt[3]{x}](https://opentextbc.ca/calculusv1openstax/wp-content/ql-cache/quicklatex.com-c3c5e7be9bc257eb2866277bae5be644_l3.png) , a office fails to be differentiable at a point where in that location is a vertical tangent line.
, a office fails to be differentiable at a point where in that location is a vertical tangent line. - As we saw with
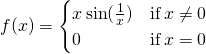 a function may neglect to be differentiable at a point in more than complicated ways besides.
a function may neglect to be differentiable at a point in more than complicated ways besides.
A Piecewise Function that is Continuous and Differentiable
Solution
For the function to be continuous at ![]() . Thus, since
. Thus, since
![]()
and ![]() , we must accept
, we must accept ![]() . Equivalently, we have
. Equivalently, we have ![]() .
.
For the function to exist differentiable at -x,
![]()
must exist. Since ![]() is defined using different rules on the right and the left, we must evaluate this limit from the right and the left and then set them equal to each other:
is defined using different rules on the right and the left, we must evaluate this limit from the right and the left and then set them equal to each other:
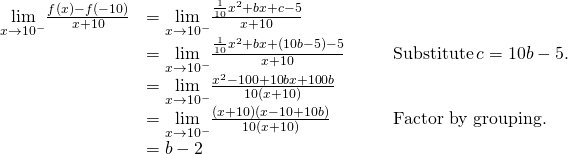
Nosotros also have

This gives us ![]() . Thus
. Thus ![]() and
and ![]() .
.
Higher-Order Derivatives
The derivative of a part is itself a function, so nosotros can find the derivative of a derivative. For example, the derivative of a position role is the rate of change of position, or velocity. The derivative of velocity is the rate of modify of velocity, which is acceleration. The new function obtained past differentiating the derivative is called the 2nd derivative. Furthermore, nosotros can continue to take derivatives to obtain the third derivative, fourth derivative, and so on. Collectively, these are referred to every bit higher-order derivatives. The notation for the college-order derivatives of ![]() tin can be expressed in whatsoever of the following forms:
tin can be expressed in whatsoever of the following forms:
![]()
![]()
![]() .
.
It is interesting to note that the notation for ![]() may be viewed as an attempt to express
may be viewed as an attempt to express ![]() more compactly. Analogously,
more compactly. Analogously, ![]() .
.
Finding a Second Derivative
For ![]() , find
, find ![]() .
.
Solution
Showtime find ![]() .
.
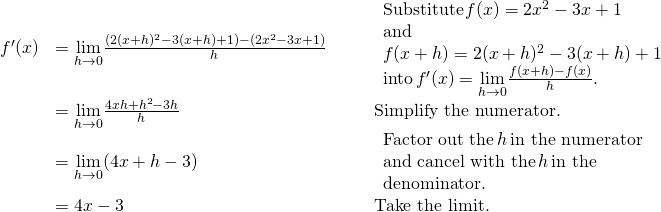
Side by side, notice ![]() past taking the derivative of
past taking the derivative of ![]() .
.
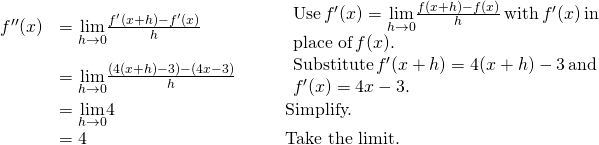
Finding Acceleration
The position of a particle along a coordinate axis at time ![]() (in seconds) is given by
(in seconds) is given by ![]() (in meters). Find the function that describes its acceleration at time
(in meters). Find the function that describes its acceleration at time ![]() .
.
Solution
Since ![]() and
and ![]() , we begin past finding the derivative of
, we begin past finding the derivative of ![]() :
:
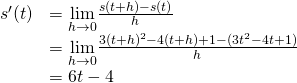
Next,
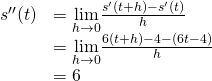
Thus, ![]() .
.
Central Concepts
Cardinal Equations
- The derivative function
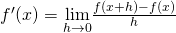
For the following exercises, utilise the definition of a derivative to find ![]() .
.
1. ![]()
2. ![]()
3. ![]()
four. ![]()
Solution
![]()
5. ![]()
6. ![]()
Solution
![]()
7. ![]()
8. ![]()
Solution
![]()
nine. ![]()
10. ![]()
Solution
![]()
For the post-obit exercises, use the graph of ![]() to sketch the graph of its derivative
to sketch the graph of its derivative ![]() .
.
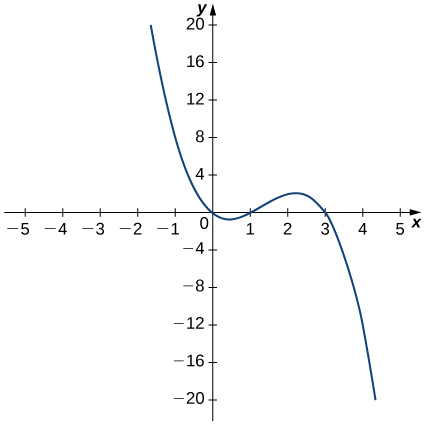
11. 
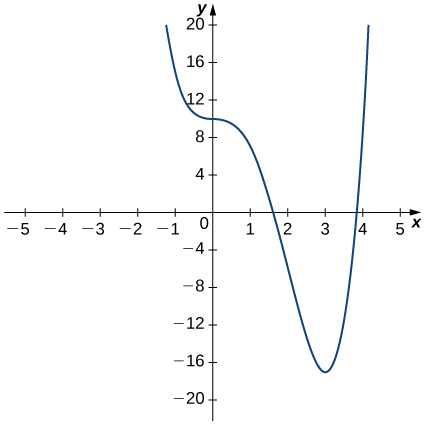
12. 
Solution

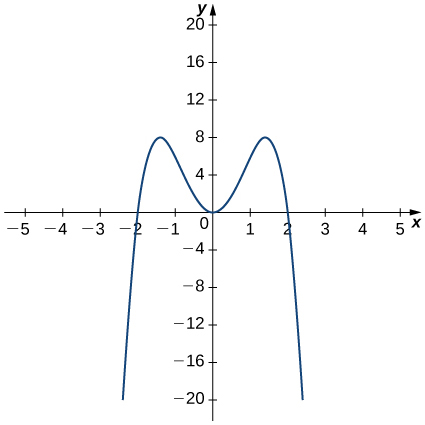
13. 
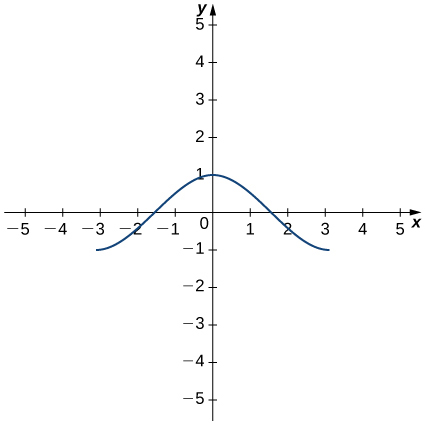
14. 
Solution

For the following exercises, the given limit represents the derivative of a part ![]() at
at ![]() . Find
. Find ![]() and
and ![]() .
.
fifteen. ![]()
16. ![]()
Solution
![]()
17. ![]()
18. ![]()
Solution
![]()
nineteen. ![]()
20. ![]()
Solution
![]()
For the following functions,
- sketch the graph and
- utilize the definition of a derivative to show that the function is not differentiable at
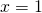 .
.
21. 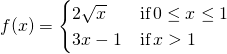
23. 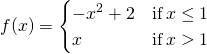
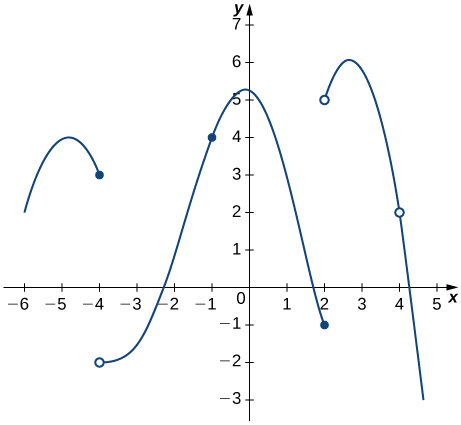
For the post-obit graphs,
- decide for which values of
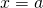 the
the  exists merely
exists merely 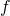 is non continuous at
is non continuous at 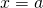 , and
, and - determine for which values of
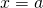 the role is continuous simply not differentiable at
the role is continuous simply not differentiable at 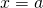 .
.
25. 
For the post-obit functions, use ![]() to find
to find ![]() .
.
28. ![]()
29. ![]()
30. ![]()
Solution
![]()
For the following exercises, use a reckoner to graph ![]() . Determine the part
. Determine the part ![]() , then utilize a calculator to graph
, then utilize a calculator to graph ![]() .
.
31. [T] ![]()
33. [T] ![]()
35. [T] ![]()
For the following exercises, describe what the 2 expressions represent in terms of each of the given situations. Be sure to include units.
37. ![]() denotes the population of a city at time
denotes the population of a city at time ![]() in years.
in years.
38. ![]() denotes the total amount of coin (in thousands of dollars) spent on concessions by
denotes the total amount of coin (in thousands of dollars) spent on concessions by ![]() customers at an amusement park.
customers at an amusement park.
Solution
a. Boilerplate charge per unit at which customers spent on concessions in thousands per customer.
b. Rate (in thousands per customer) at which ![]() customers spent coin on concessions in thousands per customer.
customers spent coin on concessions in thousands per customer.
39. ![]() denotes the total cost (in thousands of dollars) of manufacturing
denotes the total cost (in thousands of dollars) of manufacturing ![]() clock radios.
clock radios.
40. ![]() denotes the grade (in per centum points) received on a exam, given
denotes the grade (in per centum points) received on a exam, given ![]() hours of studying.
hours of studying.
Solution
a. Average grade received on the test with an average study fourth dimension between two amounts.
b. Rate (in percentage points per hour) at which the course on the test increased or decreased for a given boilerplate study time of ![]() hours.
hours.
41. ![]() denotes the cost (in dollars) of a sociology textbook at university bookstores in the United States in
denotes the cost (in dollars) of a sociology textbook at university bookstores in the United States in ![]() years since 1990.
years since 1990.
42. ![]() denotes atmospheric pressure at an altitude of
denotes atmospheric pressure at an altitude of ![]() feet.
feet.
Solution
a. Average alter of atmospheric pressure betwixt ii different altitudes.
b. Rate (torr per foot) at which atmospheric pressure is increasing or decreasing at ![]() feet.
feet.
Solution
a. The rate (in degrees per foot) at which temperature is increasing or decreasing for a given acme ![]() .
.
b. The rate of change of temperature as altitude changes at 1000 feet is -0.1 degrees per foot.
Solution
a. The rate at which the number of people who have come down with the flu is changing ![]() weeks afterward the initial outbreak.
weeks afterward the initial outbreak.
b. The rate is increasing sharply up to the third calendar week, at which betoken it slows downwards and then becomes constant.
For the post-obit exercises, use the following tabular array, which shows the height ![]() of the Saturn V rocket for the Apollo xi mission
of the Saturn V rocket for the Apollo xi mission ![]() seconds later launch.
seconds later launch.
| Time (seconds) | Height (meters) |
|---|---|
| 0 | 0 |
| 1 | 2 |
| ii | 4 |
| 3 | 13 |
| four | 25 |
| 5 | 32 |
47.What is the concrete meaning of ![]() ? What are the units?
? What are the units?
Which Of The Following Best Describes The Difference Between Data Andã¢â‚¬â€¹ Information?,
Source: https://opentextbc.ca/calculusv1openstax/chapter/the-derivative-as-a-function/
Posted by: myerstimentep.blogspot.com


0 Response to "Which Of The Following Best Describes The Difference Between Data Andã¢â‚¬â€¹ Information?"
Post a Comment